Philosophical Equivariance – Sewing Holonomies Towards Equal Trace Endomorphisms.

In d-dimensional topological field theory one begins with a category S whose objects are oriented (d − 1)-manifolds and whose morphisms are oriented cobordisms. Physicists say that a theory admits a group G as a global symmetry group if G acts on the vector space associated to each (d−1)-manifold, and the linear operator associated to each cobordism is a G-equivariant map. When we have such a “global” symmetry group G we can ask whether the symmetry can be “gauged”, i.e., whether elements of G can be applied “independently” – in some sense – at each point of space-time. Mathematically the process of “gauging” has a very elegant description: it amounts to extending the field theory functor from the category S to the category S_G whose objects are (d − 1)-manifolds equipped with a principal G-bundle, and whose morphisms are cobordisms with a G-bundle. We regard S as a subcategory of S_G by equipping each (d − 1)-manifold S with the trivial G-bundle S × G. In S_G the group of automorphisms of the trivial bundle S × G contains G, and so in a gauged theory G acts on the state space H(S): this should be the original “global” action of G. But the gauged theory has a state space H(S,P) for each G-bundle P on S: if P is non-trivial one calls H(S,P) a “twisted sector” of the theory. In the case d = 2, when S = S¹ we have the bundle P_g → S¹ obtained by attaching the ends of [0,2π] × G via multiplication by g. Any bundle is isomorphic to one of these, and P_g is isomorphic to P_g^‘ iff g′ is conjugate to g. But note that the state space depends on the bundle and not just its isomorphism class, so we have a twisted sector state space C_g = H(S,P_g) labelled by a group element g rather than by a conjugacy class.

We shall call a theory defined on the category S_G a G-equivariant Topological Field Theory (TFT). It is important to distinguish the equivariant theory from the corresponding “gauged theory”. In physics, the equivariant theory is obtained by coupling to nondynamical background gauge fields, while the gauged theory is obtained by “summing” over those gauge fields in the path integral.

An alternative and equivalent viewpoint which is especially useful in the two-dimensional case is that S_G is the category whose objects are oriented (d − 1)-manifolds S equipped with a map p : S → BG, where BG is the classifying space of G. In this viewpoint we have a bundle over the space Map(S,BG) whose fibre at p is H_p. To say that H_p depends only on the G-bundle p^∗EG on S pulled back from the universal G-bundle EG on BG by p is the same as to say that the bundle on Map(S,BG) is equipped with a flat connection allowing us to identify the fibres at points in the same connected component by parallel transport; for the set of bundle isomorphisms p^∗₀EG → p^∗₁EG is the same as the set of homotopy classes of paths from p₀ to p₁. When S = S¹ the connected components of the space of maps correspond to the conjugacy classes in G: each bundle P_g corresponds to a specific point p_g in the mapping space, and a group element h defines a specific path from p_g to p_hgh⁻¹ .

G-equivariant topological field theories are examples of “homotopy topological field theories”. Using Vladimir Turaev‘s two main results: first, an attractive generalization of the theorem that a two-dimensional TFT “is” a commutative Frobenius algebra, and, secondly, a classification of the ways of gauging a given global G-symmetry of a semisimple TFT.

Definition of the product in the G-equivariant closed theory. The heavy dot is the basepoint on S¹. To specify the morphism unambiguously we must indicate consistent holonomies along a set of curves whose complement consists of simply connected pieces. These holonomies are always along paths between points where by definition the fibre is G. This means that the product is not commutative. We need to fix a convention for holonomies of a composition of curves, i.e., whether we are using left or right path-ordering. We will take h(γ₁ ◦ γ₂) = h(γ₁) · h(γ₂).

A G-equivariant TFT gives us for each element g ∈ G a vector space C_g, associated to the circle equipped with the bundle p_g whose holonomy is g. The usual pair-of-pants cobordism, equipped with the evident G-bundle which restricts to p_g1 and p_g2 on the two incoming circles, and to p_g1g2 on the outgoing circle, induces a product

C_g1 ⊗ C_g2 → C_g1g2 —– (1)

making C := ⊕_g∈GC_g into a G-graded algebra. Also there is a trace θ: C1  → C defined by the disk diagram with one ingoing circle. The holonomy around the boundary of the disk must be 1. Making the standard assumption that the cylinder corresponds to the unit operator we obtain a non-degenerate pairing

C_g ⊗ C_g⁻¹ → C

A new element in the equivariant theory is that G acts as an automorphism group on C. That is, there is a homomorphism α : G → Aut(C) such that

α_h : C_g → C_hgh⁻¹ —– (2)

Diagramatically, α_h is defined by the surface in the immediately above figure. Now let us note some properties of α. First, if φ ∈ C_h then α_h(φ) = φ. The reason for this is diagrammatically in the below figure.

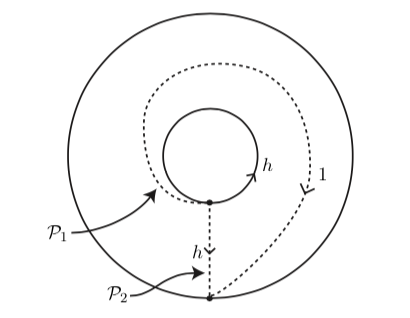
If the holonomy along path P₂ is h then the holonomy along path P₁ is 1. However, a Dehn twist around the inner circle maps P₁ into P₂. Therefore, α_h(φ) = α₁(φ) = φ, if φ ∈ C_h.

Next, while C is not commutative, it is “twisted-commutative” in the following sense. If φ₁ ∈ C_g1 and φ₂ ∈ C_g2 then

α_g2 (φ₁)φ₂ = φ₂φ₁ —– (3)

The necessity of this condition is illustrated in the figure below.

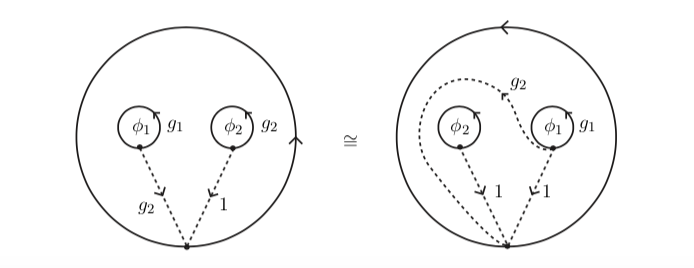
The trace of the identity map of C_g is the partition function of the theory on a torus with the bundle with holonomy (g,1). Cutting the torus the other way, we see that this is the trace of α_g on C₁. Similarly, by considering the torus with a bundle with holonomy (g,h), where g and h are two commuting elements of G, we see that the trace of α_g on C_h is the trace of α_h on C_g⁻¹. But we need a strengthening of this property. Even when g and h do not commute we can form a bundle with holonomy (g,h) on a torus with one hole, around which the holonomy will be c = hgh⁻¹g⁻¹. We can cut this torus along either of its generating circles to get a cobordism operator from C_c ⊗ C_h to C_h or from C_g⁻¹ ⊗ C_c to C_g⁻¹. If ψ ∈ C_{hgh⁻¹g⁻¹}. Let us introduce two linear transformations L_ψ, R_ψ associated to left- and right-multiplication by ψ. On the one hand, L_ψα_g : φ􏰀 ↦ ψ_αg(φ) is a map C_h → C_h. On the other hand R_ψα_h : φ ↦ α_h(φ)ψ is a map C_g⁻¹ → C_g⁻¹. The last sewing condition states that these two endomorphisms must have equal traces:

TrC_h 􏰌L_ψα_g􏰍 = TrC_g⁻¹ 􏰌R_ψα_h􏰍 —– (4)

(4) was taken by Turaev as one of his axioms. It can, however, be reexpressed in a way that we shall find more convenient. Let ∆_g ∈ C_g ⊗ C_g⁻¹ be the “duality” element corresponding to the identity cobordism of (S¹,P_g) with both ends regarded as outgoing. We have ∆_g = ∑ξ_i ⊗ ξⁱ, where ξ_i and ξⁱ ru􏰟n through dual bases of C_g and C_g⁻¹. Let us also write

∆_h = ∑η_i ⊗ ηⁱ ∈ C_h ⊗ C_h⁻¹. Then (4) is easily seen to be equivalent to

∑α_h(ξ_i)ξⁱ = 􏰟 ∑η_iα_g(ηⁱ) —– (5)

in which both sides are elements of C_{hgh⁻¹g⁻¹}.